This article demonstrates how to accurately measure the speed of light using readily available equipment and the Moku:Lab. Learn the underlying physics and experimental techniques with this comprehensive coursework.
The speed of light is a fundamental property of the universe that underlies countless areas of science and engineering. As such, having a highly accurate and precise measure of it is necessary for scientific and technological progression. Surprisingly, it is possible to get a reasonably accurate measurement without highly specialized instrumentation.
Aims
To measure the speed of light.
Learning outcomes
- Improved ability to identify limitations of an experiment in producing accurate and precise results
- Improved ability to use an oscilloscope.
- Familiarity with a phasemeter and how it works.
- Familiarity and competence with laser safety and optical equipment.
Motivation
Light travels at an extremely fast speed; however, it is finite, as Danish astronomer Ole Røemer reasoned in 1675. Røemer observed the orbits of Jupiter’s moons and noted that they appeared to speed up when the Earth was closer to Jupiter. He deduced that this change must be a result of the decreased distance the light from the moons had to travel. From this, he calculated that the speed of light was approximately 200,000 km/s.
In 1905, Albert Einstein postulated that the speed of light was constant for any observer, no matter how fast they themselves were travelling. From this he derived the theory of special relativity, interrelating both space and time and deducing the famous mass-energy equivalence equation: E=mc². Although the implications of this theory were not immediately felt, today’s society relies on it heavily for technologies such as the global positioning system (GPS).
Preliminary exercises
1. Read the background sections of “Phase 1: Time of flight method” and “Phase 2: Phase measurement method.”
2. Figure 1 shows the setup of an experiment to measure the speed of light. A laser is pulsed onto a reflector, and the time for the pulse to return back to the laser is measured using a co-located detector with some inherent system time delay tsys. The reflector is positioned first at a distance 1.5m from the detector, and a time of flight t1= 2.53 ns measured. The reflector is then moved to 1.75 m from the detector, and a time of flight t2= 4.19 ns measured. From these time measurements, calculate the speed of light:

Figure 1: Simple time flight experiment measuring the speed of light
3. Figure 2 shows the setup of an experiment to measure the speed of light. A laser is configured to output a beam of light with 10 MHz sinusoidal amplitude, and directed towards a reflector. A photodetector is co-located on the laser, and enables measurement of the phase of the reflected beam of light. The reflector is initially positioned at 1 m from the laser, and the phase of the reflected beam measured as φ1 = (0.35 * 2π) rad. The reflector is then moved to a distance 2.5 m from the detector and the phase of the reflected beam measured to be φ2 = (0.45 * 2π) rad. From these phase measurements, calculate the speed of light:
Figure 2: Simple phase measurement experiment measuring the speed of light
Phase 1: Time of flight method
Background
The speed of light can be determined using a time of flight measurement. The time of flight method of measuring the speed of light is based on the fact that the speed of light is finite and constant. Therefore, light will take a finite amount of time to travel a distance. By measuring the time the light takes to travel a certain distance, one can determine the speed in which it travelled.
Figure 3 shows the concept of a time of flight measurement. A laser beam being directed perpendicularly towards a corner cube reflector a distance d/2 away and is detected on return. Assuming the light travels at a finite speed, c, then it will take some time, t, to propagate to the corner cube reflector and back to the source (for a round trip distance of d). We can relate the propagation speed c, round trip time t, and propagation distance d with the expression c=d/t.

Figure 3: Light will take a finite amount of time, t, to travel over a distance of d
In practice, the light pulse needs to be measured using a photodetector. Figure 4 shows the setup of such a system. Light is emitted from a laser and reflected off a corner cube and is tapped off using a beam splitter and directed to a photodetector for detection.

Figure 4: Addition of a photo detector to the simplified time of flight setup
The addition of a detection system introduces a system time delay tsys component to the “time of flight” measured, tmeas. This is due to response times inherent in the detector electronics and measurement equipment.
Thus, tmeas is composed of both the system time delay tsys and true propagation time of the light pulse, t:

Theoretically, given a known propagation distance d and system time tsys, the speed of light c could be calculated with a single time of flight measurement as follows:

However it is impractical and unnecessary to measure tsys and d. By obtaining two time of flight measurements tmeas1, tmeas2 at different total propagation distances d1, d2, a differencing technique can be used to remove any systematic components of time and distance:

Thus, only the change in propagation distance Δd, and the time of flight measurements tmeas1 and tmeas2 are required to calculate c. Figure 5 depicts how the initial portion of the laser propagation path changes when the reflector is displaced by a distance Δd/2, with the effect of increasing the total propagation distance by Δd.
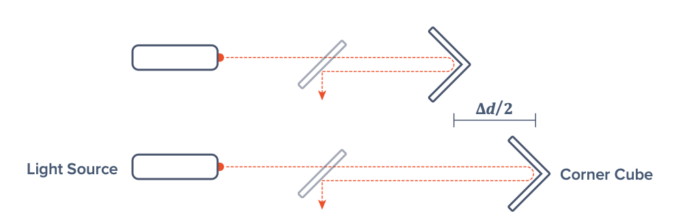
Figure 5: Reflector displacement causes a propagation time delay
In order to actually make a time of flight measurement (tmeas), the laser is pulsed, and the photo detector signal is compared with the laser’s pulsing control signal. Time measurements of the control signal will also contain some system delay; however, this is compensated for through the differencing technique.
Equipment
- Moku:Lab device
- iPad
- High-speed photo detector
- Power supply
- Beam splitter
- Focusing lens
- Corner cube reflector
- Laser
- Meter ruler
- Optical rails
Safety
Lasers can cause eye damage. Do not look directly at the laser beam when trying to align the optical components; use a piece of paper to trace the beam instead. Take care to note where the beam could be reflected to and ensure no one else is at risk of harm.
Setup
Review the experimental setup in Figure 6 below.

Figure 6: Speed of light experiment setup
Method
- Set up the equipment as in Figure 6, ensuring the power supply and Moku:Lab outputs are switched OFF. Note that the corner cube reflector is connected to the 1-meter optical rail.
- On the iPad, connect to the Moku:Lab Oscilloscope instrument (see Appendix if you are unsure of how to do this).
- On the Oscilloscope, select Input 1 and Input 2 waveforms to be viewable.
- Turn the photo detector ON using the top switch to position “|.”
- Confirm the laser is directed on the desired optical path (as shown in Figure 6).
- Ensure the power supply is turned to 0 V and 0 A before switching it ON, and then increase voltage to just above 5 V with a small amount of current to activate the laser.
- Use the Oscilloscope synthesizer to generate a 500 mVpp 30 MHz square wave on both Output 1 and Output 2 to act as a reference signal and laser amplitude control signal, respectively. Ensure the outputs are switched ON.
- Set the Oscilloscope to trigger on the rising edge of reference signal Input 2. Scale the Input 2 waveform so you can view a few square wave cycles, and adjust the trigger level as required.
- Align the laser and optical elements so the light hits the centre of the photo detector. As the corner cube will be moved throughout the experiment, it should remain aligned when slid along the entire 1-meter rail. (Hint: When aligned, the photo detector signal on Input 1 should resemble the pulsing control signal which is being viewed on Input 2 (a square wave). It is easier to align the corner cube at each end of the 1-meter rail independently. To achieve the best alignment, observe and maximize the amplitude of the photo detector signal while adjusting the optical equipment.)
- Position the corner cube so it is at one end of the optical rail (i.e., close to the beam splitter).
- On the Oscilloscope, use cursors to measure and record the time between the laser control signal rising edge (Input 2), and the rising edge of the detected beam (Input 1). Note: t = 0 should already correspond to a rising edge of Input 2 if you are triggering correctly.
- Move the corner cube to multiple different positions along the rail, noting the displacement from the initial corner cube position in Step 10. With each new position, record the time of flight as in Step 11.
- Using the equations presented in the background section, and the time of flight measurements taken at varying corner cube positions, use a line of best fit method to calculate the speed of light, c.
Questions
- How does the calculated speed of light compare to the accepted value of 299 792 458 m/s?
- What are the primary sources of error in this experiment?
- How could the accuracy be improved?
- How could the precision be improved?
Phase 2: Phase measurement method
Background
In the previous experiment the speed of light was determined by measuring the propagation delay of a pulse of light. Although this method is intuitive, there are many sources of potential errors. For instance, it is often difficult to determine the precise point in which a pulse arrives.
Another method to determine speed of light is to use the concept of phase. Phase is the position of a particular point in a waveform with respect to its wavelength. As a signal travels through space, it progresses its phase. Similar to propagation delay, the phase of a signal increases the further distance the signal travels.

Figure 7: Sine wave phase change due to changing propagation distance
Figure 7 depicts two sine (light) waves that have propagated over slightly different distances. Here, sine wave 1 has travelled some distance d and can be represented as sin(2πft). Sine wave 2, however, has traveled some distance d + Δd and now has incurred some additional phase φ, represented as sin (2πft + φ). The incurred phase φ can be related to the change in propagation distance Δd, wave frequency f and speed of light c as follows:
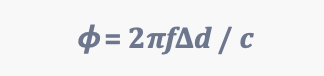
Given we know φ, f, and Δd, the speed of light, c, can be calculated:

To measure the phase of a signal, you can use the Moku:Lab Phasemeter. The Phasemeter measures the phase of a sinusoidal signal by multiplying it with another sine wave of known frequency. The following equation demonstrates the result of this multiplication:

The result is an oscillatory term, sin(4πft + φ), and a nonoscillatory term sin(φ). Oscillating terms can be removed using a low-pass filter, leaving only sin(φ). On the assumption that φ is small we can make the approximation sin(φ) ≈ φ. In practice, a feedback loop is used to ensure that φ is small.
By measuring the phase of a signal, the Phasemeter can determine the speed of light very accurately.
Equipment
Refer to equipment list of the “Phase 1: Time of flight method” section.
Safety
Lasers can cause eye damage. Do not look directly at the laser beam when trying to align the optical components; use a piece of paper to trace the beam instead. Take care to note where the beam could be reflected to and ensure no one else is at risk of harm.
Setup
Refer to Figure 6 of the “Phase 1: Time of flight method” section.
Method
- If not already done so, set up the equipment as in Figure 4 and follow steps 1-9 of “Phase 1: Time of flight method” section method to configure the equipment and align the optics.
- Open the Moku:Lab Phasemeter instrument (see Appendix if you are unsure of how to do this).
- Configure the Phasemeter to generate a 500 mVpp, 30 MHz sine wave on Output 1 and Output 2. Ensure the outputs are switched ON.
- Configure the locking frequency of both input channels to be 30 MHz.
- Position the corner cube to be at one end of the optical rail (i.e., close to the beam splitter).
- Switch to “Phase” measurement of the Phasemeter, and ensure measurements are in units of radians.
- Press “reacquire” to reset the locking loop and obtain the initial phase offset measurement. Note the Δφ value.
- Slowly slide the corner cube to multiple positions along the rail, ensuring you measure the displacement of each new position with respect to the initial position from Step 5. Record the Δφ value at each new position.
- You should observe the linear change in phase Δφ as you slide the corner cube along the rail.
Note: To avoid losing phase lock, it is important that the laser beam is not interfered with during this process. Any movement of the corner cube between positions should be slow and continuous. - Using each of the phase and displacement measurements, and the equations provided in the background section, use a line of best fit (or similar) method to calculate the speed of light.
Questions
- How does the calculated value compare with the accepted figure of 299 792 458 m/s?
- How this compare with the value computed using the Time of Flight Method in Phase 1?
- What are the primary sources of error in this approach?
- How could the accuracy be improved?
- How could the precision be improved?
- What are the practical issues with this approach?