In this post, we explore the fundamentals of Power Spectral Density (PSD) and its importance in signal analysis. See how the Moku Phasemeter can simplify component test measurements and enhance your research.
Power spectral density (PSD) is a signal’s distribution of power at different frequencies. While a time-domain analysis watches how a signal changes over time, a frequency-domain analysis like PSD examines how much of the signal’s power is present at different frequencies. For example, Figure 1 shows that Input 1 of the Moku Phasemeter has measured a spike in PSD where the signal’s power is concentrated.
When is power spectral density used?
Power spectral density allows scientists and engineers to characterize broadband, random signals. This ability is useful in signal processing applications to analyze the frequency content of signals, which helps guide the design of relevant filters. In telecommunications, the PSD enables the analysis of bandwidth usage, noise characterization, and interfering signals. PSD is also useful in radar and sonar applications to track signals and targets of interest.
What is amplitude spectral density?
Amplitude spectral density is the measure of a signal’s amplitude at different frequencies; it’s also the square root of the power spectral density.
How is power spectral density calculated?
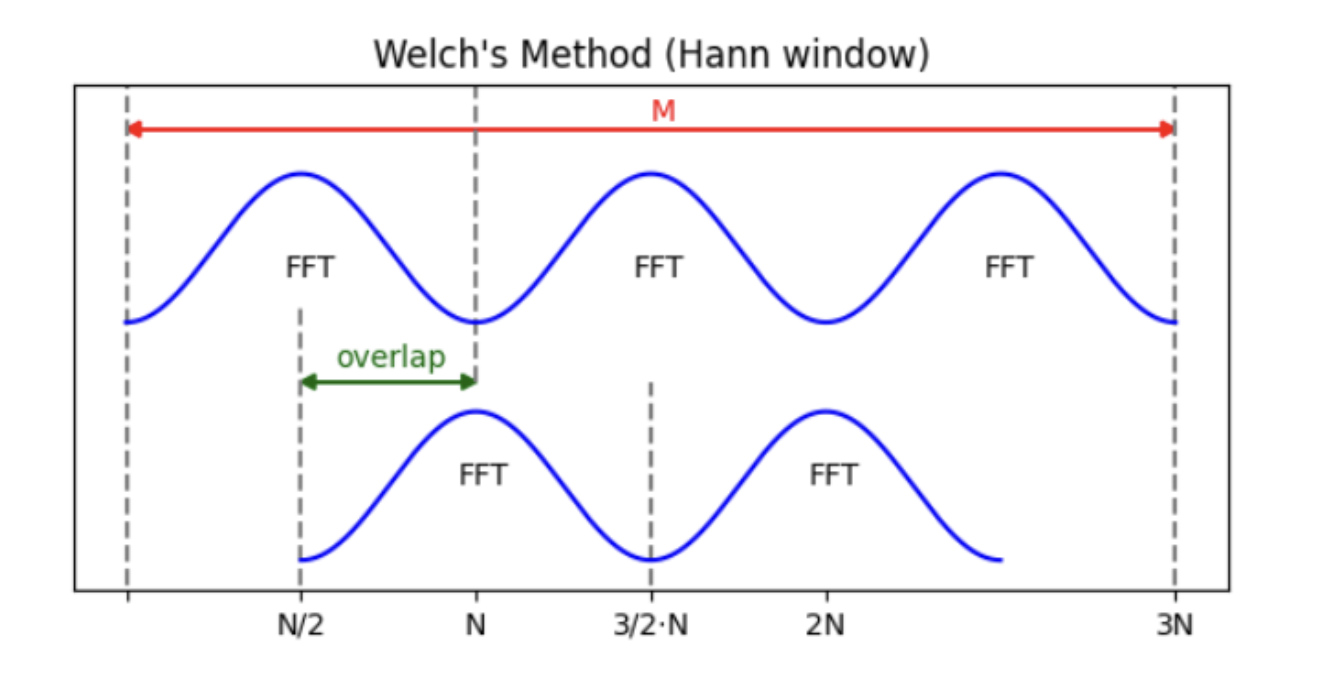
In the Moku Phasemeter, the power spectral density and amplitude spectral density are calculated using Welch’s method of overlapping periodograms, with a 50% overlap and a Hanning window. Depending on the sampling rate selected by the user, the number of points is either 512, 1024, or 2048.
This method, shown in Figure 2, is a popular non-parametric way to calculate power spectral density, using fast Fourier transform (FFT) in the analysis. An FFT allows you to convert a signal from the time domain to the frequency domain. In summary, this method takes modified periodograms of each section and averages them.
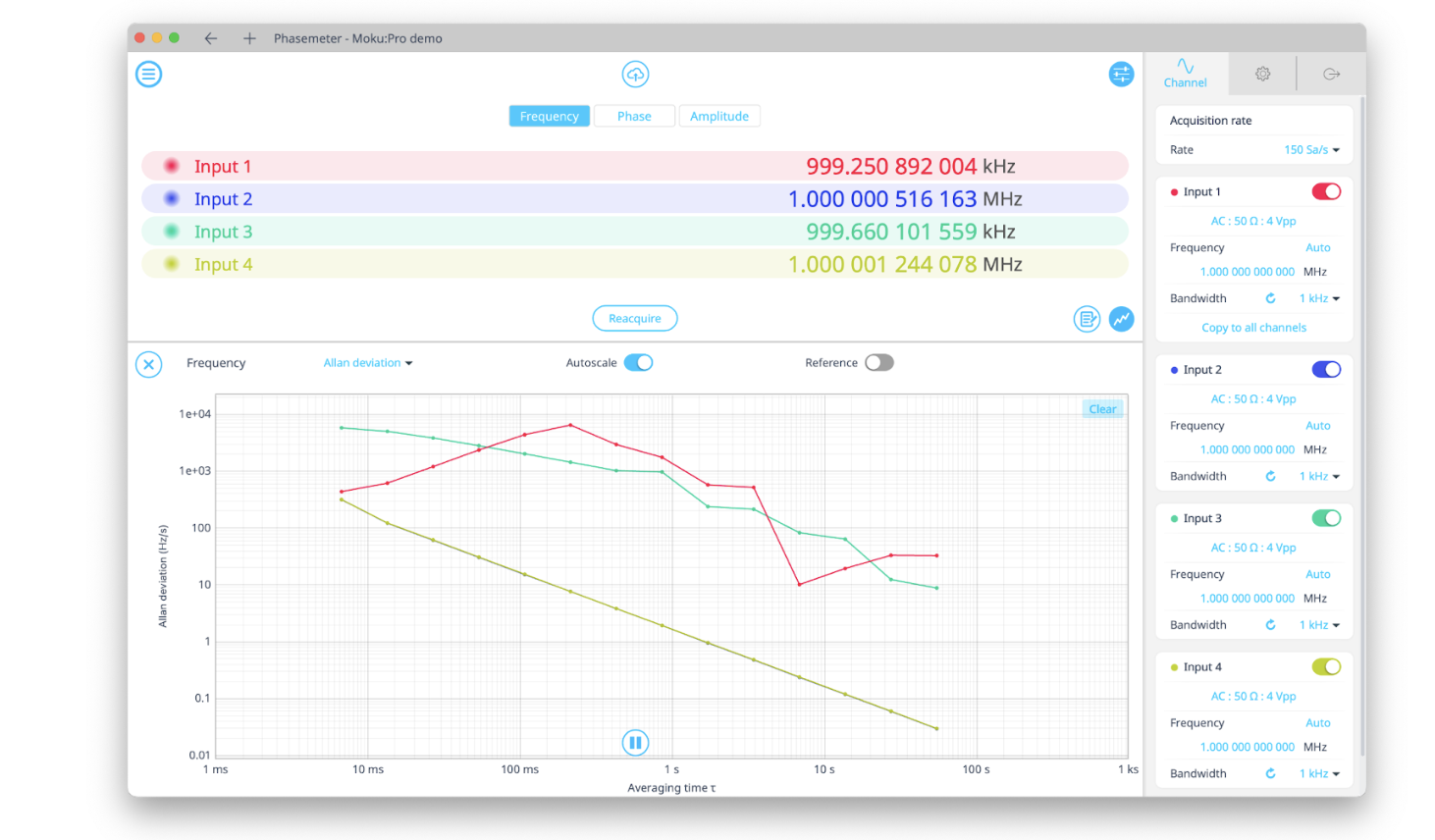
Power spectral density vs. Allan deviation
Power spectral density is the frequency domain counterpart of Allan deviation, shown in Figure 3, which is a common statistical measure to analyze time series data for applications such as oscillator characterization and is the square root of Allan variance. Both values help to describe the stability of a system.
Example: Using power spectral density for dark matter detection
Dark matter detection usually involves an experiment searching for incredibly small signals within a vast sea of noise. These signals, or events, often occur at irregular intervals over long periods of time, making the data collected from these types of experiments very challenging and time-consuming to analyze.
At Los Alamos National Laboratory, researchers used Moku:Pro to collect data, leveraging the flexible Moku Data Logger. Then, using the Moku Python API, they developed a technique to sift through these large data files in search of specific events. To detect low-amplitude events buried in noise, the team first used the Data Logger to track the background noise of the experimental setup. Then, they performed an FFT of this time-series data to determine the PSD.
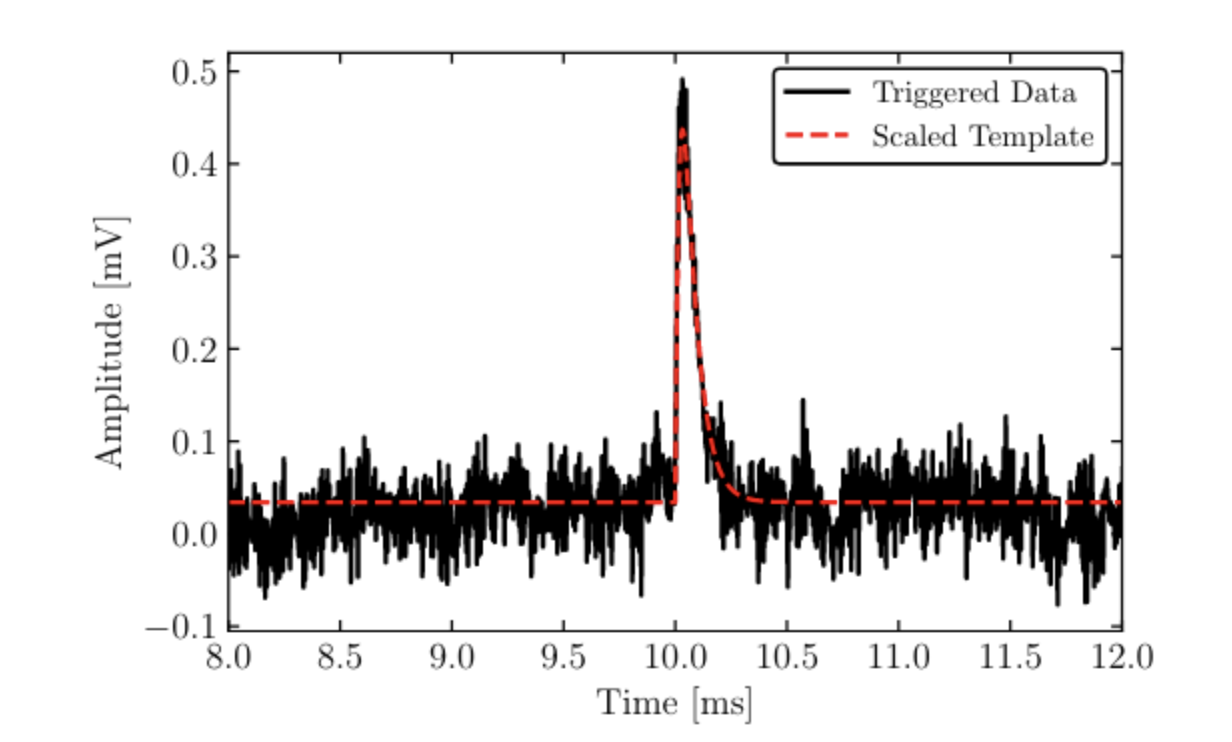
Next, the team supplied their digital acquisition software, called SPLENDAQ, as well as the PSD and an event template, resulting in the data shown in Figure 4. By using a PSD to characterize system noise, the researchers can easily detect extremely low-amplitude events.
Conclusion
PSD is a crucial tool in signal processing, allowing scientists and engineers to understand the underlying frequency content of signals. While calculating the PSD can be computationally heavy, built-in data visualization tools can reduce post-processing time and allow for better system characterization. Real-time graphs like those in the Moku Phasemeter allow engineers to view their data in parallel with the measurement.