Updated April 24, 2023.
Spectrum analyzers are essential test and measurement instruments in the lab. They are used to display and analyze signals in the frequency domain. Compared to the Fast Fourier Transform (FFT) function available in some oscilloscopes, frequency-swept spectrum analyzers typically provide better spectral resolution while maintaining a large frequency span. However, spectrum analyzers can be much slower than the FFT-based approach, especially at a finer resolution. The Moku:Lab Spectrum Analyzer instrument uses a hybrid technique, which delivers the advantages of both approaches and balances the speed and frequency span. In this guide, we will use the Moku:Lab Spectrum Analyzer and Oscilloscope to introduce the key differences between hybrid spectrum analyzer and FFT-based approaches and familiarize ourselves with some of the key settings of the Moku:Lab Spectrum Analyzer.
Limitations of FFT-based approach
Frequency domain signal analysis is an essential technique used to identify and separate unwanted noise from a signal of interest. Compared to time-domain analysis, it’s much easier to spot the noise and optimize the system to filter out the unwanted part with frequency-domain analysis. The Fast Fourier Transform is a mathematical algorithm that computes the frequency-domain responses of a signal from the time-domain trace. It is a standard built-in feature for many modern digital storage oscilloscopes and provides a good first glance of the signal in the frequency domain. However, the spectral resolution (R) of the FFT is proportional to the sampling rate (Fs) divided by the number of FFT points (N). On the other hand, the highest frequency on the FFT spectrum is limited to half the sampling rate. This means the larger the frequency span, the poorer the resolution (larger R), as demonstrated in the equations below.

This limits the FFT-based approach in characterizing finer details at high frequencies. Throughout this application note, we will use a 10 MHz sine wave, frequency modulated (FM) at 5 kHz with an FM deviation of 1 kHz as the signal of interest (Figure 1). We will use the name FM1 for this signal. Figure 2 shows the FFT spectrum of FM1, generated with Moku:Lab’s Oscilloscope. The ± 5 kHz side bands cannot be resolved by the FFT analysis. Also, multiple aliasing bands can be seen on the spectrum.

Figure 1: The FM signal at 10 MHz generated by the Moku:Lab Waveform Generator

Figure 2: FFT spectrum of the FM1, calculated by the Moku:Lab Oscilloscope
Moku:Lab hybrid Spectrum Analyzer approach
To overcome the limitation of the FFT-based approach, the Moku:Lab Spectrum Analyzer digitally mixes the signal of interest with a local oscillator (LO) f1 to bring the frequency of interest to near DC range, as shown in Figure 3. The theory of frequency mixing can be found in our Moku:Lab Lock-In Amplifier video (https://youtu.be/H2O2ADqEkHM). Then, the spectrum analyzer performs an FFT on this low frequency signal.

Figure 3: Signal processing flow of the Moku:Lab Spectrum Analyzer
As we discussed before, FFT performs best with smaller frequency spans. This hybrid approach allows the starting point of the spectral window to be selected at any given frequency (compared to the pure FFT approach, which always starts at 0). Any part of the spectrum can be analyzed with a narrow span. The spectral resolution is no longer limited by the upper frequency of the spectrum but rather is related to the frequency span. Therefore, you can get a spectrum with high resolution, even at higher frequencies. In Figure 4, we used the Moku:Lab Spectrum Analyzer to analyze FM1. All the side bands, including the significant harmonics, can be easily resolved.
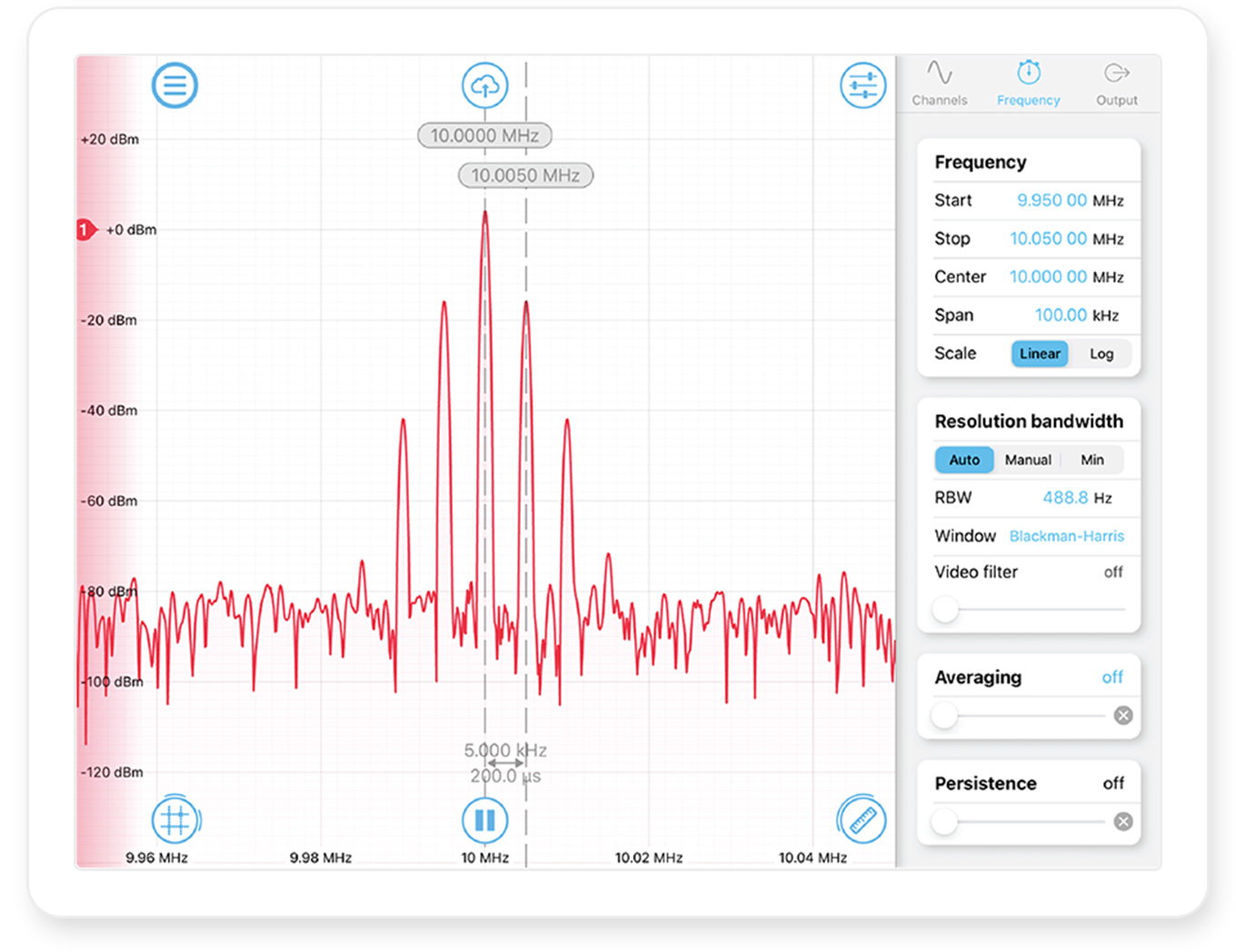
Figure 4: Spectrum of FM1, acquired with the Moku:Lab Spectrum Analyzer
Frequency, resolution bandwidth, and video filter
As we discussed in the previous section, a narrower frequency span provides finer spectral resolution. The smallest span that covers the signal frequencies of interest is recommended.
The resolution bandwidth (RBW) defines the spectral resolution of the spectrum analysis. It is determined by the number of points that are used for the FFT and the window function applied to the signal prior to calculating the FFT. A narrower resolution bandwidth provides better spectral resolution. However, it may slightly increase the measurement time.
The video filter smooths the spectrum by averaging adjacent pixels on the displayed spectrum. It is a postprocessing technique and does not affect the on-board spectrum acquisition process. A larger video filter will produce smoother spectra at the expense of poorer frequency resolution and can reduce the amplitude of narrow spectral features.
Averaging is another method to improve the signal-to-noise ratio. It has a similar effect to the video filter. It is recommended when capturing relatively stable features and does not reduce the resolution of the spectra.
Figure 5 shows the effect of resolution bandwidth, video filter, and averaging on FM1.

Figure 5: Effects of RBW, video filter, and averaging
Moku:Lab Demo mode
You can download the Moku:Lab App from the Apple App Store and experience it in demo mode. Detailed features of the Moku:Lab Spectrum Analyzer can be found at https://liquidinstruments.com/support/moku-lab-instruments/spectrum-analyzer/
Questions or comments?
Please contact us at support@liquidinstruments.com